Practical Bud Observation, Measurement and Analysis

Measurement

Manual Measurement

The path geometry that appears to conform so very well to the buds is discussed in full elsewhere.

In this section, we deal with the practical application of that geometry to real buds—or, at any rate, to photographs of them.  We discuss what measurements need to be taken, how to take them, and the difficulties that attend taking them.  We assume that our bud images are suited to the measurement process and of sufficient quality for it, as set out in the section on Observation.

We will in the first place be concerned with a bud’s profiles.  We will want to know whether or not—and if, then how well—the path geometry conforms to these profiles.

 

 

To this end, we suppose that the bud may be placed in an invariant triangle such that the tip of the bud lies at one vertex and the base of the budlies at a second.

 

 

 

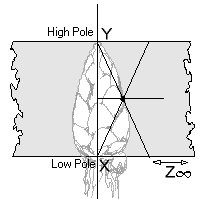
Although there is no absolute necessity that it must, the third vertex is generally taken to lie at infinity, in a direction at right angles to the line joining the first two vertices, those occupied by the tip and base of the bud.  This means that the invariant triangle presents as two, parallel lines, traversed by a third line at right angles to them—which, at first sight, may not seem to be a triangle, but is a triangle nonetheless, as it has the requisite three vertices and three sides.  In draughtsman’s terms, this triangle is a “side elevation” of the full invariant tetrahedron that we suppose pertains to our bud.  It is the side view of a tetrahedron with one real side and its two real vertices placed locally, and with its other two vertices not only at infinity, but imaginary besides.  We have an edge-on sight from infinity of (or a vertical section through) a semi-imaginary tetrahedron.

 

We usually speak of the vertices at the tip and base of the bud as the “Poles”: one of these (and either at will) may be thought of as a “source”, and the other as a “sink”, as if the path curve were arising from one, proceeding along the bud’s profile, and disappearing into the other¹.  

The path is set by measures and we want to find them, but before we set about doing so, we must first be reasonably confident that we have properly placed the poles, and when we come to attempt it, we at once find that we have a problem.

In general, it is fairly evident where the top, or high, pole should go.  The tips of most buds are usually well-defined, and easy to locate with precision, so we can for the most part be rather sure of where to put the high pole.  The same is not true of the base of the bud!  The bud attaches there to its stem, or twig, and it is seldom easy to tell where the bud stops and the twig begins.

One approach is to select a “likely looking” place for the low pole on the bud, then stick to it.

That is, we accept that the chosen place is probably not the “correct” one, but we suppose that it is “not too far” from it—and we keep it there from photo to photo, day to day.

In adopting this approach, we are in effect deciding that we are more interested in the variation with time of our
results than in their perfect precision, and our method reflects our supposition that we will see this variation best
if we keep the Inter-Pole Distance (IPD) as constant as we can.

Sometimes there is a convenient, well-defined natural mark or feature on the bud upon which we can reasonably set the low pole.  If there is no such feature (as will surely be the case with silhouettes), then, if we are working by hand on photographs or prints, we can make up a “graticule”, or template, on a slip of paper.  In fact, this is worth doing even if there is a natural mark available, as the graticule does double duty: it allows us to set off the IPD, if we need to, and to mark the heights at which our measurements are to be taken.  We place the first mark of the graticule at the tip of the bud’s image, align the graticule with the long axis, and mark the heights and the position of the low pole on the image.  It is quickly and easily done on one image after another.

As we proceed through a sequence of images, we may from time to time find reason to make a fresh graticule—the bud may be elongating, for example—but each template will be found to serve for a good many images in succession, certainly sufficiently many to justify the making of templates!

 

Also, if we are working entirely by hand, rather than with computer assistance, it can be useful to trace the outlines of the bud on tracing paper every few images.  When they are laid over other images in the same sequence, these tracings reveal changes that might otherwise go undetected, and help us to determine when a new graticule needs to be made.  If we are prepared to make pin holes in our pictures, the functions of tracing and graticule can be combined: we can use a pin to prick through the tracing paper, at the calibration marks now on the tracing paper, into the image.

 

Tracings also improve the lateral accuracy and consistency of the pole placements, as traced outline can be matched with new outline with an eye to registration.  But the holes in our pictures are permanent, and this can be a nuisance if we want to review our measurements, and perhaps make fresh ones.  We must then somehow indicate on the image which set of measurements was finally accepted.  This can be awkward.     

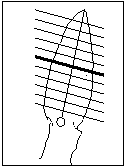
Once we have our marks we are nearly in a position to take our measurements.  We want to measure the whole widths of the bud at the marked heights, at right angles to the line joining the poles—but how are we to lay off the right angle?  We use a graduated set square and a straight edge.  The technique is as follows:

Lay the straight edge on the image, approximately parallel to the axis if the bud, and so that the entire bud remains in view.

Place the set square against the straight edge, then, on the horizontal, graduated edge of the set square, locate a graduation mark close to the bud axis.

Slide the set square back and forth along the straight edge and, while doing so, adjust the position of the straight edge until the graduation mark selected above is seen to run exactly along the bud axis—at which moment the straight edge will be accurately parallel to, and the graduated edge of the set square will be accurately at right angles to the chosen bud axis, and we can take our measurements.

While holding the straight edge firmly in the place found for it, move the set square to a height mark.

Note (that is, measure) where the left and right profiles of the bud intersect the set square at that height.

Subtract the lesser measurement from the greater.  This is the whole width of the bud at that height.

Remembering to hold the straight edge firmly in place, repeat the     previous three steps for all the height marks.

When all the height marks have been visited in this fashion, we will have our list of bud widths, and can go on to do the path curve calculations based upon them.

The accuracy of our measurements is chiefly affected by the degree of enlargement of the photographs, or print-outs: clearly, the enlargement should be as great as practically possible.  But the choice of unit is a factor, too, and that choice is affected by personal preference!  Some prefer to use inches, and tenths-of-inches.  Others prefer to use millimetres.  Those using inches report that they can measure to plus or minus about two hundredths of an inch: those using millimetres say that they manage plus or minus around one tenth of a millimetre.

The method of measurement described above is effective, but basic.  It is susceptible to all sorts of refinement:  for example, the author has incorporated a vernier calliper into a set square, with which measurements accurate to one hundredth of a millimetre were easily obtained—that is, if the image of the bud was sufficiently sharp.  Now it happens quite often that it is not, and in fact there is a practical limit to precision, set by the quality of the images, beyond which an increase in the native precision of our measuring instrument does not lead to a corresponding increase in the precision of our data.  When all is said and done and tried, the precision available from the basic arrangement depicted above is probably adequate.    

There is a basic decision to be taken about the number of heights at which we intend to take measurements.  It might be argued that we should take as many as can be physically managed, and so we would, were it not so that many of these would fall near the low pole and be measurements of twig or stem rather than of bud, and that many others might fall on local, and quite possibly spurious, imperfections.

As to that, sober reflection must present us with the thought that, even if they are basically of path curve nature, and even if no imperfections are present, the profiles of a real bud are likely to consist of variations, however slight, on a theme of “path curve”.  Discussion of the full practical and scientific implications of this is certainly in order.  This will come in the section on “Analysis”.  For now, we simply remark that multiplying the number of measurement heights eventually leads to the obliteration of useful by useless data.  In the language of the communications engineer, we eventually worsen the “signal-to-noise ratio”.

Finally, there is the question of how much time and labour can be devoted to the measurement of a single bud image.

In pre-computer days, when Lawrence Edwards (and the author) did all measurement by hand, the number of measurement heights finally settled on was seven, and this has remained the “default” even in these computerised times, though occasions do arise on which other numbers are used.

In any case, choose an odd number of heights (not including the heights of the poles).  This ensures that a measurement height falls exactly mid-way between the poles.  There are two methods of analysis in use; the so-called Projective method, developed by Lawrence Edwards, and the so-called Regression method, developed by the author.  The former requires the middle measurement height.  The latter does not, but can use it if it is there, so if we make sure that the centre height is present, both methods will be available for the same bud.

1.        It is helpful to think of a path curve like this—that is, both in terms of motion, and at one and the same moment as a whole thing, “there” in its entirety.  The motional aspect reminds us of the rhythmic transformation from which it springs, whereas the wholeness draws attention to the fact that there is a point already at every stage of the journey from source to sink.  The form of the curve stays the same: it is the invariant path that all points take.  One might think of a pilgrimage of the faithful, winding single-file along an ancient, well-beaten trail.